Eclipses and Moons - Time Differences
There is usually a difference between the Time of Maximum Eclipse and the time of the corresponding New or Full Moon. This is because the definitions of these two phenomena are based on different factors.
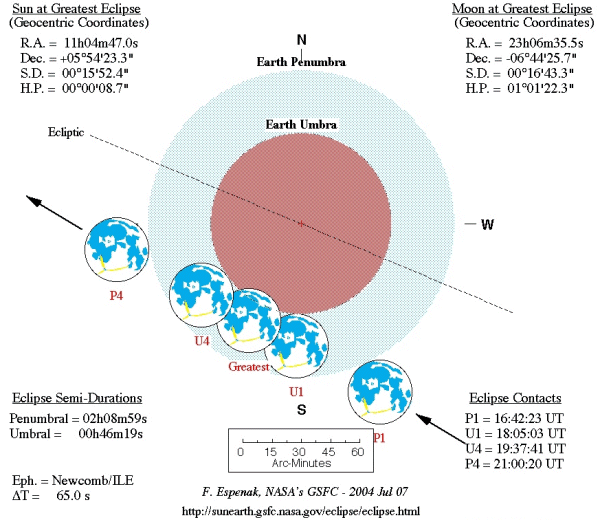
Diagram of the September 7th 2006 partial lunar eclipse, courtesy of Fred Espanak (NASA/Goddard Space Flight Center). For more information see Fred Espenak's Eclipse Home Page.
The moment of Full Moon is the moment that the Moon is exactly opposite the Sun, by longitude. You can determine this by looking up the positions of the Sun and Moon in an ephemeris. E.g., if the Full Moon is at 15° Pisces, the Sun will be exactly opposite at 15° Virgo. Note that these longitudes only take a two-dimensional picture into account, imagining the solar system on a flat plane viewed from above the Earth, as shown on any traditional astrology chart.
The moment of Maximum Eclipse, however, is based on the other dimension, as if we are standing on the Moon looking at the Earth (like the diagram above). It is the moment when the centre of the Moon is closest to the centre of the Earth's shadow (the shadow the Earth casts due to the Sun being behind it).
So, the time of Maximum Eclipse is the moment when the Moon is darkest, and in the case of a partial eclipse, the time when the biggest chunk of the Moon is in shadow.
So why do these two things not happen at the same moment?
The flat plane we look down on in an astrology chart, is shown edge-on viewed from the side in the diagram above -- it's the line through the centre of the image, the Ecliptic, i.e., the angle of the Earth's path around the Sun (or in astrology, the apparent path of the Sun around the Earth).
When viewed on a chart, the Sun, Moon and Earth look to be aligned in a straight line at Full Moon. But viewed from the side, we can see that they're not all at exactly the same height, so are not truly aligned in 3D. There's almost never a completely exact 3D alignment of the centres of the Sun, Moon and Earth, but when they're close enough to one that the Moon passes through the Earth's shadow, instead of going above or below it, we get a lunar eclipse.
In the event of a completely exact 3D alignment of the exact centres of the Sun, Moon and Earth, the time of the Full Moon and the time of Maximum Eclipse would be the same, because whichever dimension you looked from, it would hit both points simultaneously.
What usually happens though, is as in the diagram above. The large dark circle is the Earth's Umbra, which is the darkest part of the shadow the Earth casts due to the Sun being behind it. Behind the centre of that shadow is the centre of the Earth, and behind that the centre of the Sun. We are viewing from behind the Moon looking towards the Earth and Sun. The Moon is blue and white in the diagram, showing in five positions how it will move past the Earth during that lunar eclipse, from bottom right to top left.
You can see that the Moon is passing slightly below the Earth's shadow, and comes in at an angle (the Moon orbits at a slight angle to the ecliptic). Due to this angle, the Moon first aligns with the centre of the Earth by longitude, as viewed from a right angle above the ecliptic (Full Moon position) and shortly afterwards reaches the point where the maximum amount of Moon is in the shadow (Maximum Eclipse position, shown in the diagram as the middle one of the five Moons).
So the Maximum Eclipse is a few minutes away from the Full Moon, due to the Moon's declination and the fact that the eclipse is not a completely exact 3D alignment. Even a Total Eclipse is unlikely to be 100% exact.
Obviously, similar principles apply to solar eclipses, but they occur at New Moon, Sun conjunct Moon. For these, the time of Maximum Eclipse is defined as the time the centre of the Moon's shadow (which sweeps across the Earth during the eclipse) is closest to aligning with the centre of the Earth.
My Eclipse Finder here, gives timings for moments of Maximum Eclipse, and the Moon page here gives timings for New and Full Moons.

The moment of Full Moon is the moment that the Moon is exactly opposite the Sun, by longitude. You can determine this by looking up the positions of the Sun and Moon in an ephemeris. E.g., if the Full Moon is at 15° Pisces, the Sun will be exactly opposite at 15° Virgo. Note that these longitudes only take a two-dimensional picture into account, imagining the solar system on a flat plane viewed from above the Earth, as shown on any traditional astrology chart.
The moment of Maximum Eclipse, however, is based on the other dimension, as if we are standing on the Moon looking at the Earth (like the diagram above). It is the moment when the centre of the Moon is closest to the centre of the Earth's shadow (the shadow the Earth casts due to the Sun being behind it).
So, the time of Maximum Eclipse is the moment when the Moon is darkest, and in the case of a partial eclipse, the time when the biggest chunk of the Moon is in shadow.
So why do these two things not happen at the same moment?
The flat plane we look down on in an astrology chart, is shown edge-on viewed from the side in the diagram above -- it's the line through the centre of the image, the Ecliptic, i.e., the angle of the Earth's path around the Sun (or in astrology, the apparent path of the Sun around the Earth).
When viewed on a chart, the Sun, Moon and Earth look to be aligned in a straight line at Full Moon. But viewed from the side, we can see that they're not all at exactly the same height, so are not truly aligned in 3D. There's almost never a completely exact 3D alignment of the centres of the Sun, Moon and Earth, but when they're close enough to one that the Moon passes through the Earth's shadow, instead of going above or below it, we get a lunar eclipse.
In the event of a completely exact 3D alignment of the exact centres of the Sun, Moon and Earth, the time of the Full Moon and the time of Maximum Eclipse would be the same, because whichever dimension you looked from, it would hit both points simultaneously.
What usually happens though, is as in the diagram above. The large dark circle is the Earth's Umbra, which is the darkest part of the shadow the Earth casts due to the Sun being behind it. Behind the centre of that shadow is the centre of the Earth, and behind that the centre of the Sun. We are viewing from behind the Moon looking towards the Earth and Sun. The Moon is blue and white in the diagram, showing in five positions how it will move past the Earth during that lunar eclipse, from bottom right to top left.
You can see that the Moon is passing slightly below the Earth's shadow, and comes in at an angle (the Moon orbits at a slight angle to the ecliptic). Due to this angle, the Moon first aligns with the centre of the Earth by longitude, as viewed from a right angle above the ecliptic (Full Moon position) and shortly afterwards reaches the point where the maximum amount of Moon is in the shadow (Maximum Eclipse position, shown in the diagram as the middle one of the five Moons).
So the Maximum Eclipse is a few minutes away from the Full Moon, due to the Moon's declination and the fact that the eclipse is not a completely exact 3D alignment. Even a Total Eclipse is unlikely to be 100% exact.
Obviously, similar principles apply to solar eclipses, but they occur at New Moon, Sun conjunct Moon. For these, the time of Maximum Eclipse is defined as the time the centre of the Moon's shadow (which sweeps across the Earth during the eclipse) is closest to aligning with the centre of the Earth.
My Eclipse Finder here, gives timings for moments of Maximum Eclipse, and the Moon page here gives timings for New and Full Moons.